How To Find Atomic Mass Unit Of An Element
two.3: Calculating Atomic Masses
- Page ID
- 98688
Skills to Develop
- Define the atomic mass unit and average atomic mass
- Summate boilerplate atomic mass and isotopic affluence
- Ascertain the amount unit mole and the related quantity Avogadro'southward number
- Explain the relation between mass, moles, and numbers of atoms or molecules, and perform calculations deriving these quantities from 1 another
Video \(\PageIndex{1}\): A review of counting subatomic particles and a preview of isotopes and relative atomic mass.
Isotopes
The symbol for a specific isotope of any element is written by placing the mass number as a superscript to the left of the element symbol (Figure \(\PageIndex{4}\)). The atomic number is sometimes written as a subscript preceding the symbol, just since this number defines the element'due south identity, as does its symbol, it is oftentimes omitted. For example, magnesium exists equally a mixture of three isotopes, each with an atomic number of 12 and with mass numbers of 24, 25, and 26, respectively. These isotopes can be identified equally 24Mg, 25Mg, and 26Mg. These isotope symbols are read as "element, mass number" and can be symbolized consistent with this reading. For instance, 24Mg is read equally "magnesium 24," and can be written every bit "magnesium-24" or "Mg-24." 25Mg is read as "magnesium 25," and can be written as "magnesium-25" or "Mg-25." All magnesium atoms have 12 protons in their nucleus. They differ only because a 24Mg atom has 12 neutrons in its nucleus, a 25Mg atom has xiii neutrons, and a 26Mg has 14 neutrons.
Figure \(\PageIndex{1}\): The symbol for an atom indicates the element via its usual ii-letter symbol, the mass number as a left superscript, the atomic number as a left subscript (sometimes omitted), and the accuse equally a right superscript.
Information about the naturally occurring isotopes of elements with atomic numbers ane through 10 is given in Table \(\PageIndex{2}\). Notation that in addition to standard names and symbols, the isotopes of hydrogen are oft referred to using common names and accompanying symbols. Hydrogen-two, symbolized twoH, is too called deuterium and sometimes symbolized D. Hydrogen-3, symbolized 3H, is also called tritium and sometimes symbolized T.
| Element | Symbol | Atomic Number | Number of Protons | Number of Neutrons | Mass (amu) | % Natural Abundance |
|---|---|---|---|---|---|---|
| hydrogen | \(\ce{^1_1H}\) (protium) | 1 | ane | 0 | 1.0078 | 99.989 |
| \(\ce{^2_1H}\) (deuterium) | i | ane | i | 2.0141 | 0.0115 | |
| \(\ce{^3_1H}\) (tritium) | 1 | 1 | ii | three.01605 | — (trace) | |
| helium | \(\ce{^3_2He}\) | 2 | two | 1 | 3.01603 | 0.00013 |
| \(\ce{^4_2He}\) | ii | 2 | 2 | four.0026 | 100 | |
| lithium | \(\ce{^6_3Li}\) | three | 3 | 3 | half-dozen.0151 | 7.59 |
| \(\ce{^7_3Li}\) | three | 3 | 4 | 7.0160 | 92.41 | |
| beryllium | \(\ce{^9_4Be}\) | iv | 4 | 5 | nine.0122 | 100 |
| boron | \(\ce{^{x}_5B}\) | 5 | 5 | 5 | 10.0129 | 19.9 |
| \(\ce{^{11}_5B}\) | 5 | five | six | eleven.0093 | lxxx.1 | |
| carbon | \(\ce{^{12}_6C}\) | six | half-dozen | half dozen | 12.0000 | 98.89 |
| \(\ce{^{13}_6C}\) | 6 | 6 | 7 | 13.0034 | one.eleven | |
| \(\ce{^{14}_6C}\) | 6 | 6 | 8 | 14.0032 | — (trace) | |
| nitrogen | \(\ce{^{14}_7N}\) | 7 | 7 | 7 | 14.0031 | 99.63 |
| \(\ce{^{fifteen}_7N}\) | 7 | 7 | 8 | 15.0001 | 0.37 | |
| oxygen | \(\ce{^{sixteen}_8O}\) | 8 | viii | 8 | 15.9949 | 99.757 |
| \(\ce{^{17}_8O}\) | eight | viii | ix | 16.9991 | 0.038 | |
| \(\ce{^{18}_8O}\) | 8 | 8 | 10 | 17.9992 | 0.205 | |
| fluorine | \(\ce{^{nineteen}_9F}\) | ix | 9 | 10 | 18.9984 | 100 |
| neon | \(\ce{^{20}_{ten}Ne}\) | ten | 10 | 10 | nineteen.9924 | xc.48 |
| \(\ce{^{21}_{x}Ne}\) | 10 | 10 | 11 | 20.9938 | 0.27 | |
| \(\ce{^{22}_{10}Ne}\) | 10 | x | 12 | 21.9914 | 9.25 |
Utilise this Build an Atom simulator to build atoms of the first 10 elements, see which isotopes be, check nuclear stability, and gain experience with isotope symbols.
Diminutive Mass
Because each proton and each neutron contribute approximately i amu to the mass of an atom, and each electron contributes far less, the atomic mass of a single atom is approximately equal to its mass number (a whole number). Nevertheless, the average masses of atoms of most elements are non whole numbers because most elements exist naturally as mixtures of two or more isotopes.
The mass of an chemical element shown in a periodic tabular array or listed in a table of diminutive masses is a weighted, average mass of all the isotopes present in a naturally occurring sample of that element. This is equal to the sum of each individual isotope's mass multiplied by its fractional affluence.
\[\mathrm{average\: mass}=\sum_{i}(\mathrm{partial\: abundance\times isotopic\: mass})_i\]
For instance, the element boron is composed of ii isotopes: About 19.9% of all boron atoms are xB with a mass of 10.0129 amu, and the remaining lxxx.1% are xiB with a mass of xi.0093 amu. The average atomic mass for boron is calculated to be:
\[\begin{align*}
\textrm{boron average mass} &=\mathrm{(0.199\times10.0129\: amu)+(0.801\times11.0093\: amu)}\\
&=\mathrm{1.99\: amu+8.82\: amu}\\
&=\mathrm{10.81\: amu}
\finish{align*}\]
Information technology is important to sympathize that no single boron atom weighs exactly x.8 amu; 10.eight amu is the boilerplate mass of all boron atoms, and individual boron atoms weigh either approximately 10 amu or 11 amu.
Instance \(\PageIndex{1}\): Adding of Average Atomic Mass
A meteorite plant in central Indiana contains traces of the noble gas neon picked up from the solar wind during the meteorite'due south trip through the solar organization. Assay of a sample of the gas showed that it consisted of 91.84% xxNe (mass nineteen.9924 amu), 0.47% 21Ne (mass 20.9940 amu), and 7.69% 22Ne (mass 21.9914 amu). What is the average mass of the neon in the solar current of air?
Solution
\[\begin{align*}
\mathrm{average\: mass} &=\mathrm{(0.9184\times19.9924\: amu)+(0.0047\times20.9940\: amu)+(0.0769\times21.9914\: amu)}\\
&=\mathrm{(eighteen.36+0.099+1.69)\:amu}\\
&=\mathrm{20.15\: amu}
\cease{align*}\]
The average mass of a neon atom in the solar wind is 20.15 amu. (The average mass of a terrestrial neon atom is 20.1796 amu. This result demonstrates that nosotros may find slight differences in the natural abundance of isotopes, depending on their origin.)
Exercise \(\PageIndex{1}\)
A sample of magnesium is found to incorporate 78.seventy% of 24Mg atoms (mass 23.98 amu), ten.xiii% of 25Mg atoms (mass 24.99 amu), and 11.17% of 26Mg atoms (mass 25.98 amu). Calculate the boilerplate mass of a Mg atom.
- Answer
-
24.31 amu
We can also practise variations of this blazon of calculation, equally shown in the next example.
Example \(\PageIndex{2}\): Calculation of Percentage Abundance
Naturally occurring chlorine consists of 35Cl (mass 34.96885 amu) and 37Cl (mass 36.96590 amu), with an boilerplate mass of 35.453 amu. What is the percent limerick of Cl in terms of these 2 isotopes?
Solution
The average mass of chlorine is the fraction that is 35Cl times the mass of 35Cl plus the fraction that is 37Cl times the mass of 37Cl.
\[\mathrm{average\: mass=(fraction\: of\: ^{35}Cl\times mass\: of\: ^{35}Cl)+(fraction\: of\: ^{37}Cl\times mass\: of\: ^{37}Cl)}\]
If nosotros let 10 represent the fraction that is 35Cl, and so the fraction that is 37Cl is represented by 1.00 − x.
(The fraction that is 35Cl + the fraction that is 37Cl must add together up to i, so the fraction of 37Cl must equal 1.00 − the fraction of 35Cl.)
Substituting this into the average mass equation, we have:
\[\begin{align*}
\mathrm{35.453\: amu} &=(10\times 34.96885\: \ce{amu})+[(1.00-ten)\times 36.96590\: \ce{amu}]\\
35.453 &=34.96885x+36.96590-36.96590x\\
1.99705x &=one.513\\
x&=\dfrac{ane.513}{1.99705}=0.7576
\end{marshal*}\]
Then solving yields: x = 0.7576, which means that i.00 − 0.7576 = 0.2424. Therefore, chlorine consists of 75.76% 35Cl and 24.24% 37Cl.
Exercise \(\PageIndex{2}\)
Naturally occurring copper consists of 63Cu (mass 62.9296 amu) and 65Cu (mass 64.9278 amu), with an average mass of 63.546 amu. What is the per centum composition of Cu in terms of these 2 isotopes?
- Answer
-
69.15% Cu-63 and 30.85% Cu-65
Employ this simulator to make mixtures of the main isotopes of the first xviii elements, gain experience with average diminutive mass, and check naturally occurring isotope ratios.
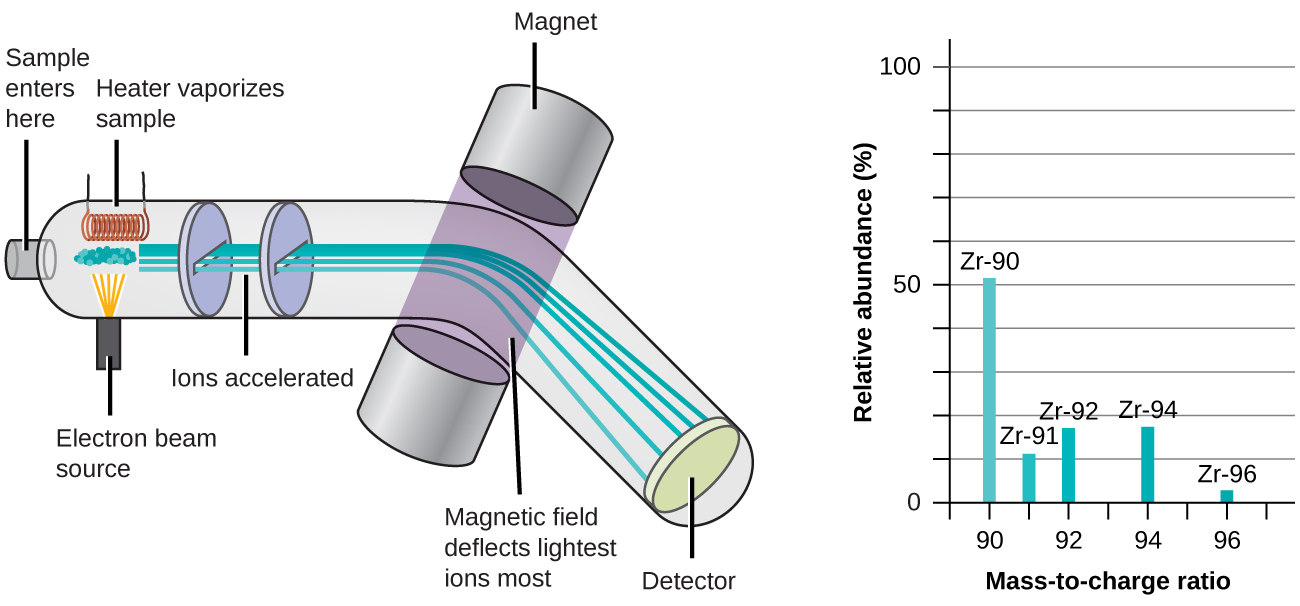
Figure \(\PageIndex{2}\): Analysis of zirconium in a mass spectrometer produces a mass spectrum with peaks showing the different isotopes of Zr.
The occurrence and natural abundances of isotopes can exist experimentally determined using an instrument called a mass spectrometer. Mass spectrometry (MS) is widely used in chemical science, forensics, medicine, environmental science, and many other fields to analyze and assistance identify the substances in a sample of cloth. In a typical mass spectrometer (Figure \(\PageIndex{5}\)), the sample is vaporized and exposed to a high-energy electron axle that causes the sample'due south atoms (or molecules) to go electrically charged, typically by losing one or more electrons. These cations then pass through a (variable) electric or magnetic field that deflects each cation's path to an extent that depends on both its mass and accuse (similar to how the path of a big steel ball begetting rolling by a magnet is deflected to a lesser extent that that of a pocket-size steel BB). The ions are detected, and a plot of the relative number of ions generated versus their mass-to-charge ratios (a mass spectrum) is made. The height of each vertical feature or top in a mass spectrum is proportional to the fraction of cations with the specified mass-to-accuse ratio. Since its initial utilize during the development of modern atomic theory, MS has evolved to get a powerful tool for chemical analysis in a wide range of applications.
Video \(\PageIndex{ii}\): Scout this video from the Royal Society for Chemical science for a brief description of the rudiments of mass spectrometry.
The Mole
The identity of a substance is divers not only past the types of atoms or ions it contains, but by the quantity of each type of atom or ion. For example, water, HiiO, and hydrogen peroxide, H2O2, are akin in that their respective molecules are composed of hydrogen and oxygen atoms. However, because a hydrogen peroxide molecule contains two oxygen atoms, equally opposed to the h2o molecule, which has simply one, the ii substances showroom very unlike properties. Today, we possess sophisticated instruments that let the direct measurement of these defining microscopic traits; nonetheless, the same traits were originally derived from the measurement of macroscopic properties (the masses and volumes of bulk quantities of thing) using relatively simple tools (balances and volumetric glassware). This experimental arroyo required the introduction of a new unit for amount of substances, the mole, which remains indispensable in modern chemical science.
The mole is an amount unit similar to familiar units like pair, dozen, gross, etc. Information technology provides a specific mensurate of the number of atoms or molecules in a majority sample of affair. A mole is defined as the amount of substance containing the same number of discrete entities (such as atoms, molecules, and ions) as the number of atoms in a sample of pure 12C weighing exactly 12 chiliad. I Latin connotation for the word "mole" is "large mass" or "bulk," which is consequent with its use as the proper noun for this unit. The mole provides a link between an easily measured macroscopic belongings, majority mass, and an extremely important fundamental property, number of atoms, molecules, and and then forth.
The number of entities composing a mole has been experimentally determined to be \(6.02214179 \times 10^{23}\), a fundamental constant named Avogadro'due south number (NA ) or the Avogadro constant in honor of Italian scientist Amedeo Avogadro. This constant is properly reported with an explicit unit of "per mole," a conveniently rounded version beingness \(half-dozen.022 \times ten^{23}/\ce{mol}\).
Video \(\PageIndex{iii}\): What is Avogadro'south Number?
Consistent with its definition every bit an amount unit, 1 mole of any element contains the same number of atoms as ane mole of whatsoever other element. The masses of 1 mole of different elements, however, are dissimilar, since the masses of the individual atoms are drastically different. The molar mass of an chemical element (or compound) is the mass in grams of 1 mole of that substance, a belongings expressed in units of grams per mole (yard/mol) (Figure \(\PageIndex{three}\)).
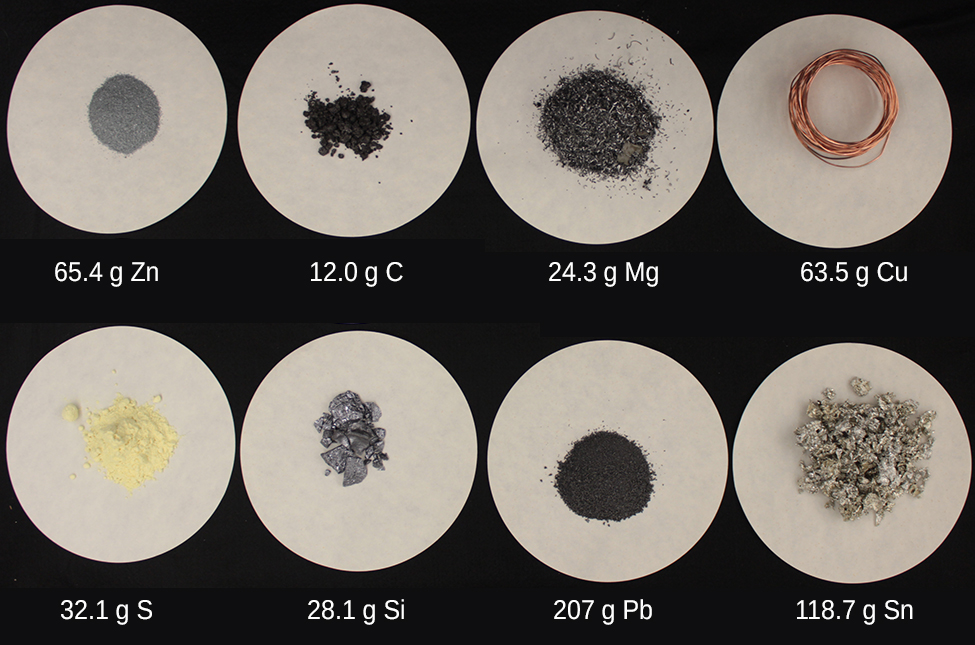
Figure \(\PageIndex{3}\): Each sample contains \(6.022 \times 10^{23}\) atoms —1.00 mol of atoms. From left to right (peak row): 65.4 thousand zinc, 12.0 g carbon, 24.three g magnesium, and 63.5 one thousand copper. From left to right (bottom row): 32.1 g sulfur, 28.1 g silicon, 207 g pb, and 118.7 thousand tin can. (credit: modification of work past Mark Ott).
Because the definitions of both the mole and the atomic mass unit are based on the same reference substance, 12C, the tooth mass of any substance is numerically equivalent to its atomic or formula weight in amu. Per the amu definition, a single 12C atom weighs 12 amu (its atomic mass is 12 amu). The erstwhile definition of the mole was that a mole was 12 thou of 12C contains 1 mole of 12C atoms (its tooth mass is 12 grand/mol). This human relationship holds for all elements, since their diminutive masses are measured relative to that of the amu-reference substance, 12C. Extending this principle, the molar mass of a compound in grams is likewise numerically equivalent to its formula mass in amu (Effigy \(\PageIndex{4}\)). On May 20, 2019 the definition was permanently changed to Avogadro's number: a mole is \(6.02214179 \times x^{23}\) of any object, from atoms to apples.1

Effigy \(\PageIndex{4}\): Each sample contains \(6.022 \times 10^{23}\) molecules or formula units—one.00 mol of the chemical compound or element. Clock-wise from the upper left: 130.ii g of C8H17OH (ane-octanol, formula mass 130.2 amu), 454.four g of HgI2 (mercury(II) iodide, formula mass 454.4 amu), 32.0 g of CH3OH (methanol, formula mass 32.0 amu) and 256.5 one thousand of South8 (sulfur, formula mass 256.5 amu). (credit: Sahar Atwa).
| Element | Average Atomic Mass (amu) | Tooth Mass (grand/mol) | Atoms/Mole |
|---|---|---|---|
| C | 12.01 | 12.01 | \(6.022 \times 10^{23}\) |
| H | 1.008 | 1.008 | \(6.022 \times 10^{23}\) |
| O | 16.00 | xvi.00 | \(vi.022 \times 10^{23}\) |
| Na | 22.99 | 22.99 | \(6.022 \times 10^{23}\) |
| Cl | 33.45 | 35.45 | \(6.022 \times 10^{23}\) |
While atomic mass and tooth mass are numerically equivalent, proceed in mind that they are vastly unlike in terms of calibration, as represented by the vast divergence in the magnitudes of their respective units (amu versus g). To capeesh the enormity of the mole, consider a small drop of water weighing virtually 0.03 g (see Figure \(\PageIndex{5}\)). Although this represents just a tiny fraction of 1 mole of water (~eighteen g), it contains more water molecules than tin can exist clearly imagined. If the molecules were distributed every bit among the roughly seven billion people on earth, each person would receive more than 100 billion molecules.

Figure \(\PageIndex{5}\): The number of molecules in a single droplet of water is roughly 100 billion times greater than the number of people on earth. (credit: "tanakawho"/Wikimedia eatables)
Video \(\PageIndex{four}\): The mole is used in chemistry to correspond \(6.022 \times ten^{23}\) of something, but it can be difficult to conceptualize such a large number. Watch this video and then complete the "Think" questions that follow. Explore more about the mole by reviewing the data under "Dig Deeper."
The relationships betwixt formula mass, the mole, and Avogadro'southward number can exist applied to compute various quantities that draw the composition of substances and compounds. For case, if we know the mass and chemical composition of a substance, nosotros tin can make up one's mind the number of moles and calculate number of atoms or molecules in the sample. Likewise, if we know the number of moles of a substance, we can derive the number of atoms or molecules and calculate the substance's mass.
Example \(\PageIndex{iii}\): Deriving Moles from Grams for an Chemical element
According to nutritional guidelines from the US Department of Agriculture, the estimated average requirement for dietary potassium is 4.seven 1000. What is the estimated boilerplate requirement of potassium in moles?
Solution
The mass of K is provided, and the respective amount of Thou in moles is requested. Referring to the periodic table, the diminutive mass of K is 39.ten amu, then its molar mass is 39.10 g/mol. The given mass of K (four.7 g) is a bit more than than 1-tenth the molar mass (39.ten g), so a reasonable "ballpark" estimate of the number of moles would exist slightly greater than 0.1 mol.
The molar amount of a substance may be calculated by dividing its mass (grand) past its molar mass (g/mol):

The factor-characterization method supports this mathematical arroyo since the unit "1000" cancels and the answer has units of "mol:"
\[ \mathrm{iv.7\; \abolish{yard} Thousand \left ( \dfrac{mol\; K}{39.10\;\abolish{g}}\right)=0.12\;mol\; K} \nonumber\]
The calculated magnitude (0.12 mol K) is consistent with our ballpark expectation, since it is a bit greater than 0.ane mol.
Practice \(\PageIndex{3}\): Beryllium
Glucinium is a light metal used to fabricate transparent 10-ray windows for medical imaging instruments. How many moles of Be are in a thin-foil window weighing 3.24 g?
- Respond
-
0.360 mol
Case \(\PageIndex{4}\): Deriving Grams from Moles for an Element
A liter of air contains \(9.2 \times 10^{−4}\) mol argon. What is the mass of Ar in a liter of air?
Solution
The tooth amount of Ar is provided and must be used to derive the corresponding mass in grams. Since the amount of Ar is less than 1 mole, the mass will exist less than the mass of i mole of Ar, approximately 40 chiliad. The molar amount in question is approximately one-one thousandth (~x−3) of a mole, and then the corresponding mass should be roughly i-one thousandth of the molar mass (~0.04 one thousand):

In this case, logic dictates (and the gene-label method supports) multiplying the provided amount (mol) by the molar mass (m/mol):
\[\mathrm{9.two \times10^{-4}\; \cancel{mol} \; Ar \left( \dfrac{39.95\;g}{\cancel{mol}\;Ar} \right)=0.037\;chiliad\; Ar} \nonumber\]
The result is in agreement with our expectations, around 0.04 m Ar.
Exercise \(\PageIndex{4}\)
What is the mass of 2.561 mol of golden?
- Respond
-
504.4 g
Example \(\PageIndex{6}\): Deriving Number of Atoms from Mass for an Element
Copper is commonly used to fabricate electrical wire (Effigy \(\PageIndex{6}\)). How many copper atoms are in v.00 g of copper wire?

Figure \(\PageIndex{6}\): Copper wire is composed of many, many atoms of Cu. (credit: Emilian Robert Vicol)
Solution
The number of Cu atoms in the wire may be conveniently derived from its mass by a two-step computation: first calculating the molar amount of Cu, and then using Avogadro's number (NA ) to catechumen this tooth amount to number of Cu atoms:

Considering that the provided sample mass (v.00 thou) is a little less than 1-tenth the mass of i mole of Cu (~64 g), a reasonable estimate for the number of atoms in the sample would be on the club of ane-tenth NA , or approximately 1022 Cu atoms. Conveying out the two-step computation yields:
\[\mathrm{5.00\:\cancel{g}\:Cu\left(\dfrac{\cancel{mol}\:Cu}{63.55\:\cancel{g}}\right)\left(\dfrac{6.022\times10^{23}\:atoms}{\abolish{mol}}\right)=four.74\times10^{22}\:atoms\: of\: copper}\]
The factor-label method yields the desired cancellation of units, and the computed upshot is on the gild of 1022 equally expected.
Exercise \(\PageIndex{half-dozen}\)
A prospector panning for gilt in a river collects 15.00 grand of pure gold. How many Au atoms are in this quantity of gilded?
- Respond
-
\(4.586 \times 10^{22}\; Au\) atoms
Example \(\PageIndex{seven}\): Deriving Moles from Grams for a Compound
Our bodies synthesize protein from amino acids. One of these amino acids is glycine, which has the molecular formula CtwoH5OtwoN. How many moles of glycine molecules are contained in 28.35 g of glycine?
Solution
We tin derive the number of moles of a compound from its mass following the same procedure nosotros used for an chemical element in Case \(\PageIndex{6}\):

The molar mass of glycine is required for this calculation, and it is computed in the same fashion as its molecular mass. One mole of glycine, CtwoHvO2Northward, contains 2 moles of carbon, v moles of hydrogen, two moles of oxygen, and 1 mole of nitrogen:
The provided mass of glycine (~28 g) is a bit more than than i-third the molar mass (~75 one thousand/mol), then we would expect the computed result to be a bit greater than i-3rd of a mole (~0.33 mol). Dividing the compound'south mass by its molar mass yields:
\[\mathrm{28.35\:\cancel{grand}\:glycine\left(\dfrac{mol\: glycine}{75.07\:\cancel{g}}\right)=0.378\:mol\: glycine} \nonumber\]
This result is consistent with our crude estimate.
Exercise \(\PageIndex{7}\)
How many moles of sucrose, \(C_{12}H_{22}O_{11}\), are in a 25-grand sample of sucrose?
- Respond
-
0.073 mol
Example \(\PageIndex{8}\): Deriving Grams from Moles for a Compound
Vitamin C is a covalent compound with the molecular formula C6H8Osix. The recommended daily dietary allowance of vitamin C for children aged four–viii years is 1.42
Solution
Equally for elements, the mass of a compound can exist derived from its tooth amount as shown:

The molar mass for this chemical compound is computed to exist 176.124 g/mol. The given number of moles is a very small fraction of a mole (~10−4 or one-ten thousandth); therefore, we would expect the corresponding mass to exist well-nigh 1-ten thousandth of the molar mass (~0.02 g). Performing the adding, we become:
\[\mathrm{1.42\times10^{-4}\:\cancel{mol}\:vitamin\: C\left(\dfrac{176.124\:k}{\cancel{mol}\:vitamin\: C}\right)=0.0250\:m\: vitamin\: C} \nonumber\]
This is consistent with the anticipated outcome.
Exercise \(\PageIndex{8}\)
What is the mass of 0.443 mol of hydrazine, \(N_2H_4\)?
- Respond
-
14.two g
Instance \(\PageIndex{9}\): Deriving the Number of Molecules from the Compound Mass
A package of an artificial sweetener contains 40.0 mg of saccharin (C7H5NOiiiS), which has the structural formula:
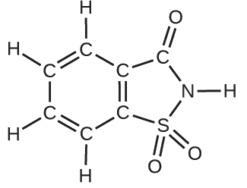
Given that saccharin has a molar mass of 183.18 thou/mol, how many saccharin molecules are in a 40.0-mg (0.0400-g) sample of saccharin? How many carbon atoms are in the same sample?
Solution
The number of molecules in a given mass of compound is computed by beginning deriving the number of moles, as demonstrated in Instance \(\PageIndex{8}\), and then multiplying by Avogadro'southward number:

Using the provided mass and molar mass for saccharin yields:
\[\mathrm{0.0400\:\cancel{yard}\:\ce{C7H5NO3S}\left(\dfrac{\abolish{mol}\:\ce{C7H5NO3S}}{183.eighteen\:\cancel{g}\:\ce{C7H5NO3S}}\right)\left(\dfrac{six.022\times10^{23}\:\ce{C7H5NO3S}\:molecules}{1\:\abolish{mol}\:\ce{C7H5NO3S}}\right)}\\
=\mathrm{ane.31\times10^{twenty}\:\ce{C7H5NO3S}\:molecules}\]
The chemical compound'due south formula shows that each molecule contains seven carbon atoms, and and so the number of C atoms in the provided sample is:
\[\mathrm{1.31\times10^{xx}\:\ce{C7H5NO3S}\: molecules\left(\dfrac{7\:C\: atoms}{1\:\ce{C7H5NO3S}\: molecule}\right)=9.20\times10^{21}\:C\: atoms} \nonumber\]
Practice \(\PageIndex{9}\)
How many \(C_4H_{ten}\) molecules are contained in 9.213 g of this compound? How many hydrogen atoms?
- Answer
-
- \(9.545 \times ten^{22}\; \text{molecules}\; C_4H_{10}\)
- \(nine.545 \times 10^{23 }\;\text{atoms}\; H\)
Video \(\PageIndex{5}\): A preview of some of the uses we will have for moles in upcoming units
Summary
Video \(\PageIndex{6}\): Watch this video for a review of relative diminutive mass and isotopes.
An atom consists of a pocket-sized, positively charged nucleus surrounded by electrons. The nucleus contains protons and neutrons; its bore is about 100,000 times smaller than that of the atom. The mass of one cantlet is commonly expressed in atomic mass units (amu), which is referred to every bit the atomic mass. An amu is defined as exactly \(1/12\) of the mass of a carbon-12 cantlet and is equal to 1.6605 \(\times\) x−24 g.
Protons are relatively heavy particles with a charge of i+ and a mass of 1.0073 amu. Neutrons are relatively heavy particles with no charge and a mass of 1.0087 amu. Electrons are low-cal particles with a charge of 1− and a mass of 0.00055 amu. The number of protons in the nucleus is called the atomic number (Z) and is the property that defines an atom's elemental identity. The sum of the numbers of protons and neutrons in the nucleus is called the mass number and, expressed in amu, is approximately equal to the mass of the atom. An atom is neutral when information technology contains equal numbers of electrons and protons.
Isotopes of an element are atoms with the same atomic number but unlike mass numbers; isotopes of an element, therefore, differ from each other only in the number of neutrons within the nucleus. When a naturally occurring element is equanimous of several isotopes, the atomic mass of the element represents the average of the masses of the isotopes involved. A chemical symbol identifies the atoms in a substance using symbols, which are ane-, ii-, or iii-letter abbreviations for the atoms.
How Sciences Interconnect
Counting Neurotransmitter Molecules in the Brain
The brain is the control center of the central nervous organization (Effigy \(\PageIndex{7}\)). It sends and receives signals to and from muscles and other internal organs to monitor and control their functions; it processes stimuli detected by sensory organs to guide interactions with the external world; and information technology houses the complex physiological processes that requite ascension to our intellect and emotions. The broad field of neuroscience spans all aspects of the structure and function of the central nervous system, including enquiry on the beefcake and physiology of the brain. Great progress has been made in brain research over the past few decades, and the Encephalon Initiative, a federal initiative announced in 2013, aims to advance and capitalize on these advances through the concerted efforts of various industrial, academic, and regime agencies (more details available at world wide web.whitehouse.gov/share/brain-initiative).
Figure \(\PageIndex{vii}\):(a) A typical human encephalon weighs nigh 1.5 kg and occupies a volume of roughly 1.one L. (b) Information is transmitted in encephalon tissue and throughout the primal nervous arrangement by specialized cells called neurons (micrograph shows cells at 1600× magnification).
Specialized cells chosen neurons transmit data between different parts of the central nervous system by way of electrical and chemical signals. Chemical signaling occurs at the interface between different neurons when i of the cells releases molecules (called neurotransmitters) that lengthened across the small gap between the cells (called the synapse) and bind to the surface of the other cell. These neurotransmitter molecules are stored in small intracellular structures called vesicles that fuse to the cell wall and then break open to release their contents when the neuron is appropriately stimulated. This process is called exocytosis (see Effigy \(\PageIndex{eight}\)). One neurotransmitter that has been very extensively studied is dopamine, C8H11NO2. Dopamine is involved in various neurological processes that touch on a wide variety of human behaviors. Dysfunctions in the dopamine systems of the encephalon underlie serious neurological diseases such as Parkinson'due south and schizophrenia.
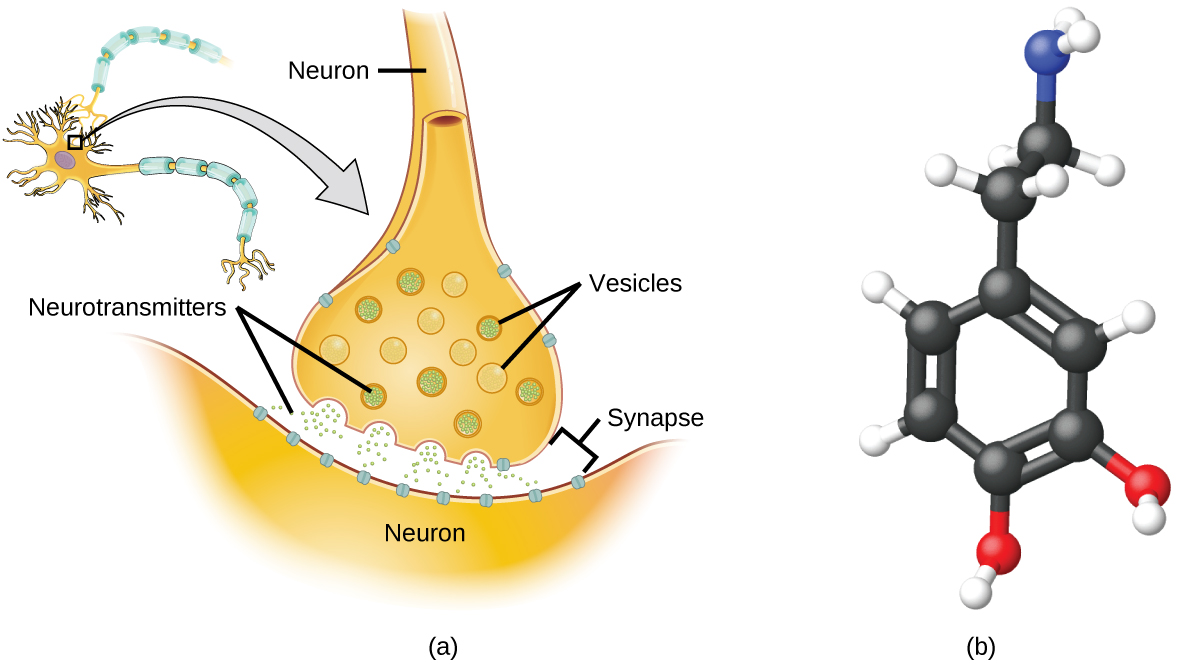
Figure \(\PageIndex{8}\): (a) Chemical signals are transmitted from neurons to other cells by the release of neurotransmitter molecules into the small gaps (synapses) betwixt the cells. (b) Dopamine, C8H11NO2, is a neurotransmitter involved in a number of neurological processes.
One important aspect of the complex processes related to dopamine signaling is the number of neurotransmitter molecules released during exocytosis. Since this number is a cardinal cistron in determining neurological response (and subsequent human being idea and activeness), information technology is of import to know how this number changes with certain controlled stimulations, such every bit the administration of drugs. It is likewise of import to understand the mechanism responsible for whatever changes in the number of neurotransmitter molecules released—for example, some dysfunction in exocytosis, a change in the number of vesicles in the neuron, or a change in the number of neurotransmitter molecules in each vesicle.
Significant progress has been made recently in directly measuring the number of dopamine molecules stored in private vesicles and the amount really released when the vesicle undergoes exocytosis. Using miniaturized probes that can selectively notice dopamine molecules in very small amounts, scientists take determined that the vesicles of a certain blazon of mouse brain neuron incorporate an average of 30,000 dopamine molecules per vesicle (about 5×10−twenty mol or fifty zmol). Analysis of these neurons from mice subjected to various drug therapies shows significant changes in the boilerplate number of dopamine molecules independent in individual vesicles, increasing or decreasing by up to 3-fold, depending on the specific drug used. These studies as well indicate that not all of the dopamine in a given vesicle is released during exocytosis, suggesting that it may be possible to regulate the fraction released using pharmaceutical therapies.2
Looking Beyond
Video \(\PageIndex{7}\): Call back our exploration into the size of an cantlet final calendar week? This video goes deeper into investigating the size of the subatomic particles we just discussed.
Footnotes
1. Read more about the redefinition of SI units including the kilogram here (Laura Howe, CE&N, Nov. sixteen, 2018).
2. Omiatek, Donna M., Amanda J. Bressler, Ann-Sofie Cans, Anne K. Andrews, Michael L. Heien, and Andrew G. Ewing. "The Real Catecholamine Content of Secretory Vesicles in the CNS Revealed past Electrochemical Cytometry."Scientific Report 3 (2013): 1447, accessed January 14, 2015, doi:10.1038/srep01447.
Key Equations
- \(\mathrm{average\: mass}=\sum_{i}(\mathrm{fractional\: abundance \times isotopic\: mass})_i\)
Glossary
- anion
- negatively charged atom or molecule (contains more electrons than protons)
- atomic mass
- average mass of atoms of an element, expressed in amu
- diminutive mass unit (amu)
- (too, unified atomic mass unit, u, or Dalton, Da) unit of mass equal to \(\dfrac{1}{12}\) of the mass of a 12C atom
- atomic number (Z)
- number of protons in the nucleus of an atom
- cation
- positively charged cantlet or molecule (contains fewer electrons than protons)
- chemical symbol
- 1-, 2-, or 3-letter abbreviation used to represent an element or its atoms
- Dalton (Da)
- culling unit equivalent to the atomic mass unit of measurement
- fundamental unit of charge
- (also called the elementary charge) equals the magnitude of the charge of an electron (e) with e = 1.602 \(\times\) 10−19 C
- ion
- electrically charged atom or molecule (contains unequal numbers of protons and electrons)
- mass number (A)
- sum of the numbers of neutrons and protons in the nucleus of an atom
- mole
- amount of substance containing the aforementioned number of atoms, molecules, ions, or other entities equally the number of atoms in exactly 12 grams of 12C
- molar mass
- mass in grams of 1 mole of a substance
- unified atomic mass unit (u)
- alternative unit equivalent to the atomic mass unit
Contributors
-
Paul Flowers (Academy of Due north Carolina - Pembroke), Klaus Theopold (Academy of Delaware) and Richard Langley (Stephen F. Austin State Academy) with contributing authors.Textbook content produced past OpenStax College is licensed under a Artistic Commons Attribution License iv.0 license. Download for free at http://cnx.org/contents/85abf193-2bd...a7ac8df6@9.110).
- Adelaide Clark, Oregon Constitute of Technology
- Fuse School, Open up Educational Resource gratuitous of charge, nether a Creative Commons License: Attribution-NonCommercial CC BY-NC (View License Deed: https://creativecommons.org/licenses/by-nc/iv.0/)
- Crash Course Chemistry, Crash Form is a sectionalisation of Complexly and videos are free to stream for educational purposes.
- TED-Ed's delivery to creating lessons worth sharing is an extension of TED'due south mission of spreading great ideas. Inside TED-Ed'due south growing library of TED-Ed animations, you will notice advisedly curated educational videos, many of which represent collaborations between talented educators and animators nominated through the TED-Ed website.
Feedback
Take feedback to requite about this text? Click here.
Constitute a typo and desire actress credit? Click hither.
Source: https://chem.libretexts.org/Courses/Oregon_Institute_of_Technology/OIT%3A_CHE_201_-_General_Chemistry_I_(Anthony_and_Clark)/Unit_2%3A_The_Structure_of_the_Atom/2.3%3A_Calculating_Atomic_Masses
Posted by: fullerondowde.blogspot.com


0 Response to "How To Find Atomic Mass Unit Of An Element"
Post a Comment